Difference between revisions of "NumericalDiffEqs"
| Line 1: | Line 1: | ||
| + | == Equilibrium Potentials == | ||
| + | |||
| + | You can find the equilibrium potential for given channel conductances. Set the injected current to zero I = 0. Then fix and absorb the gating variables and maximal conductances <math> (g_L, g_{Na}, g_{K}) </math> into single conductance variables <math> (G_L, G_{Na}, G_{K}) </math>. | ||
| + | |||
| + | For example | ||
| + | |||
| + | <math> G_{K} = n^4 g_K </math> | ||
| + | |||
| + | Then the differential equation for the membrane is | ||
| + | |||
| + | <math> C \frac{dV}{dt} = - G_L (V - E_L) - G_{Na} (V - E_{Na}) - G_K (V - E_K) </math> | ||
| + | |||
| + | The equilibrium potential is the value of V such that <math> \frac{dV}{dt} </math> is zero. You can plug in <math> \frac{dV}{dt} = 0 </math> and solve for <math> V_{equilibrium}. </math> | ||
| + | |||
| + | <math> V_{equilibrium} = \frac{G_L E_L + G_{Na} E_{Na} + G_K E_K}{G_L + G_{Na} + G_K} </math> | ||
| + | |||
| + | The equilibrium potential is the weighted average of the reversal potentials -- weighted by the corresponding conductances. Note that the weights add to one. | ||
| + | |||
| + | == Numerical Solution of Differential Equations == | ||
| + | |||
Remember the equation for the cell with only leak channels. | Remember the equation for the cell with only leak channels. | ||
Revision as of 23:37, 12 February 2009
Equilibrium Potentials
You can find the equilibrium potential for given channel conductances. Set the injected current to zero I = 0. Then fix and absorb the gating variables and maximal conductances 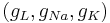 into single conductance variables
into single conductance variables 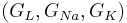 .
.
For example
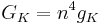
Then the differential equation for the membrane is
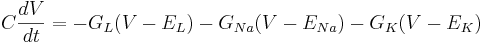
The equilibrium potential is the value of V such that 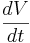 is zero. You can plug in
is zero. You can plug in 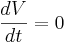 and solve for
and solve for 
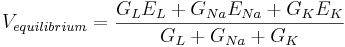
The equilibrium potential is the weighted average of the reversal potentials -- weighted by the corresponding conductances. Note that the weights add to one.
Numerical Solution of Differential Equations
Remember the equation for the cell with only leak channels.

Let's simplify: suppose there is no injected current and that the reversal potential for the leak channels is 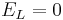 . Then our equation is
. Then our equation is
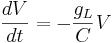
Using different letters for the variables (because this is done in the software linked below):
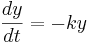
Here k is the rate constant, 1/k is the time constant, 1/k is 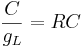 in the notation above. A leaky cell is what is called an RC circuit -- a resistor and capacitor together in a circuit. The time constant of an RC circuit is RC. The bigger k, the higher the rate of convergence, and the smaller the time constant 1/k. The time constant is the time it takes the solution to decay to 1/e of its value.
in the notation above. A leaky cell is what is called an RC circuit -- a resistor and capacitor together in a circuit. The time constant of an RC circuit is RC. The bigger k, the higher the rate of convergence, and the smaller the time constant 1/k. The time constant is the time it takes the solution to decay to 1/e of its value.
Solution of differential equations happens at discrete times: 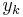 , separated by small time intervals dt.
, separated by small time intervals dt.
The simplest way of solving this equation is with Euler's method:
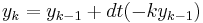
This is a special case of the general formula for Euler's method applied to the (vector) differential equation
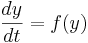

Click here for code for visualizing the numerical solution of differential equations.