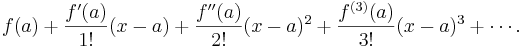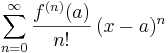Difference between revisions of "Taylor Series"
From Sean_Carver
(→Questions for Exploration in Groups of Three (With Computer)) |
(→Questions for Exploration in Groups of Three (With Computer)) |
||
| Line 53: | Line 53: | ||
* What is imprecise about the previous question? Why should it make a mathematician cringe? | * What is imprecise about the previous question? Why should it make a mathematician cringe? | ||
| + | |||
| + | Instead of cos(x) try the function | ||
| + | |||
| + | <math> f(x) = \frac{1}{x^2+1} </math> | ||
Revision as of 23:39, 14 March 2011
Contents
Review Concepts
- Sequences
- Convergence
- Infinite series
- The sequence of partial sums of an infinite series
- Power series
New Concept: Taylor Series
- Think of Taylor series as a special kind of power series, where the sequence of partial sums are meant as better and better approximations of some other function.
- The Taylor Series is derived from the function.
Definition copied, verified, and adapted from Wikipedia, this page (permanent link). See license to copy, modify, distribute.
The Taylor series of a function ƒ(x) at a is the power series
which can be written in the more compact sigma notation as
- Note that for nth approximate partial sum, the only information used about the function
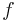 , is its derivatives at
, is its derivatives at 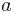 up to order
up to order 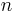 .
. - Convergence: A function whose Taylor series converges to the function is called analytic. But sometimes, as we have seen with power series generally, convergence can occur only within a radius of convergence.
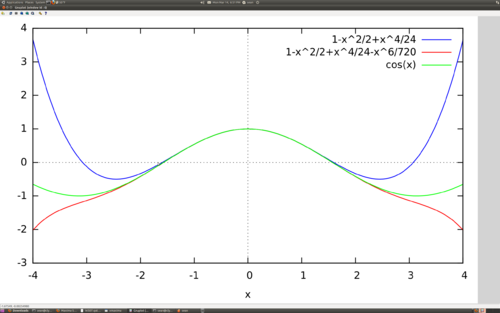
- Example: The Taylor series for cos(x), at a = 0. Partial sums for 4 and 6 terms.
Try it yourself
Open Maxima and type (or copy and paste):
a:0; n1:4; n2:6; M:4; plot2d([taylor(cos(x),x,a,n1), taylor(cos(x),x,a,n2), cos(x)],[x,-M,M]);
After you have typed in the above you can change the parameters a, n1, n2, M by scrolling up and changing them, then pressing return.
-
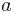 is where the derivatives are taken
is where the derivatives are taken - n1 and n2 are the orders for the two taylor series plots
- [-M, M] is the range of the plot
Questions for Exploration in Groups of Three (With Computer)
- Does the series seem to converge in the range [-M,M] =[-8,8]? If so, how large should n1 or n2 be so that this convergence is apparent?
- What is imprecise about the previous question? Why should it make a mathematician cringe?
Instead of cos(x) try the function